Dendrochronology and Archaeoastronomy

Karl-Heinz Lewin

Dendrochronology

The Swedish couple, Petra Ossowski Larsson & Lars-Åke Larsson, operate the software company Cybis Electronics & Data AB. Incidentally, the two engage in research into local history and came to dendrochronology with the aim of determining the exact construction year of some wooden houses in the Stockholm archipelago. The problem there lies in synchronizing individual tree-trunks with each other, or with mean-value curves obtained from many tree-trunks, while considering the individually different growth conditions of the trees. To facilitate the time-consuming task, Larsson developed two programs: CDendro, that analyzes and compares the obtained data with several established statistical methods and helps to find ‘matching’ synchronization points; and CooRecorder, that semi-automatically measures the ring-widths from images of tree sections that had been scanned with a flat-bed scanner or photographed, and stores the results in a file format that can be processed by CDendro [1]. The programs are distributed to dendrochronologists and other prospective markets.

From as many ‘samples’ as possible, i.e. individual trunks of the same species and from the same region, each of which should have at least 100 year-rings, a reference chronology can be built. Older trees are inserted into the already existing measurement series, when their ring-width data have as many overlaps with it as possible, and have exactly one significant point of synchronization with it, which is determined with several different statistical methods to increase reliability. (The directions and the amounts of the variations of tree-ring widths of one tree, or of an average sequence derived from a collection of trees, form a pattern. A tree-ring sequence A is said to “synchronize” with another tree-ring sequence B at a given tree-ring S in that sequence, when the last ring of A is positioned at S in B, and the patterns of the two sequences in the overlapping portions of the two sequences match according to a selected statistical algorithm.) An important role in the synchronization of samples or chronologies with each other (known in English as ‘cross-dating’) is played by the so-called t-test [2]. The t value should here be preferably greater than 6, as smaller t values can lead to multiple and therefore not unique significant synchronization points, and they often do so [3]. The two Larssons collected tree samples and created their own reference chronology, and were now able to date wooden houses. At the same time their software was tested and improved.

After the Larssons gained access to the raw data of a Finnish pine chronology more than 7,600 years long, with more than 1,500 sample trees, which had been compiled from currently felled trees and then trees from lakes and swamps, they were able to confirm the correctness of the data of this published chronology with their software. They could also demonstrate that the 1,800 years long West Danish oak chronology, with end-date of 1986, had exactly one significant synchronous position with the Finnish pine chronology just in that year [4]. A prerequisite for the synchronization between chronologies from different tree species from different regions is that they each consist of several hundred individual measuring series, and that one can find a clear and unique significant synchronizing position [1].

Dendrochronology and Phantom Time

In 2013, Ossowski Larsson wrote:

About this time, I heard of Illig’s hypothesis [see C&C Review 2015:3, pp. 23-24] on a trip from Hamburg to Denmark (Illig has never been discussed in Sweden). I looked it up on the Internet and then ordered his books. Totally insane idea, but two things caught my interest: the singularity of the Palatine Chapel [i.e. Aachen Cathedral’s Octagon] and the three-days too short [Gregorian] calendar reform. The thesis of the fictitious years should be denied or confirmed by dendrochronology. [5]

With their software the Larssons had the tool to begin the task. They started with the Irish oak chronology, following Freedom of Information litigation in 2010 which forced Queen’s University Belfast (QUB) to make the raw data for their 7,000 years of Irish-English oak chronology publicly available. The Larssons chose only Irish samples and included only those with more than 100 year-rings and sorted the data by localities, chose one as a starting point and started to build a chronology. After two months of working, with the support of their son Hans-Petter, they had produced three assured chronologies, which they called ‘BelfastAD’, ‘LateBC’ and ‘BelfastLong’, and which agreed almost exactly with the partial chronologies identified by Michael Bailllie from QUB. BelfastAD started with living trees, spanning the period from 2006 back to 25 CE (Common Era, i.e. our Era); BelfastLong and LateBC were dated by Baillie, after cross-dating with English oak finds, to the years 5452 to 837 BCE (Before CE) and 1155 to 69 BCE, respectively. These latter two partial chronologies are “floating” [6].

BelfastLong itself also contains a gap of 75 years around 2450 BCE. This gap was safely bridged with data from an English oak collection from a peat-bog near Croston (1442 years with large overlaps on both sides, correlation coefficient (cc) 0.33, t-value (t) 12.9). But although BelfastLong and LateBC overlap by 318 years, they match only badly (cc 0.17, t 3.0). Later the Larssons identified an English sample collection from Swan Carr that establishes the overlap and fits well to LateBC (cc 0.30, t 8.0), but not to BelfastLong. Without this collection BelfastLong and LateBC do not fit together (cc 0.10, t 1.0), even with an overlap of 108 years. Also, attempts to bridge the gap with data from recent tree finds succeeded only on one side [7].

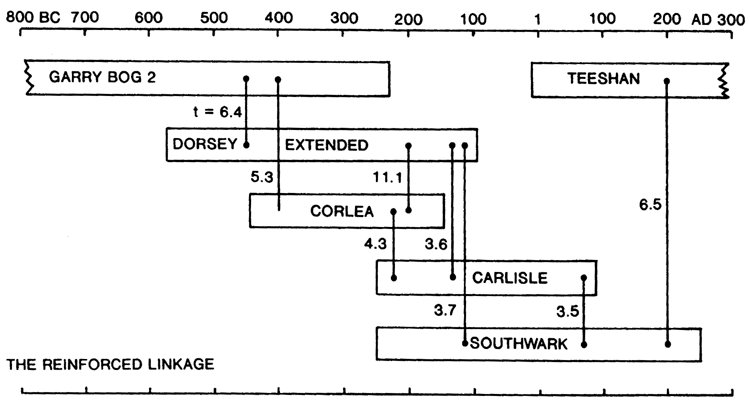
More important in our context is the gap between LateBC and BelfastAD. Baillie bridged this gap with Roman wood from Carlisle (Northern England) and Southwark, from the Roman shipyard at Londinium. According to Baillie, Southwark fits well with Teeshan in BelfastAD, with 250 years of overlap and t = 6.5, but both Carlisle and Southwark show only weak connections to the LateBC localities as well as to each other with t values around 4 (see fig. 1). The Larssons received the raw data from Cathy Tyers, Sheffield University / English Heritage, and found that Carlisle does not fit to BelfastAD, but Southwark fits well to SouthEnglishRoman and thus to LateBC, but not to BelfastAD, contrary to Baillie’s description [7]! Robert M. Porter uses this finding of the Larssons in two articles, one in conference proceedings [8] and another in the journal of the SIS [9], to indicate problems of dendrochronology, and considered Baillie’s solution as “far from certain” [10]. How then could Baillie date so accurately? Ossowski Larsson cites a report of QUB from 1983 which refers to ‘wiggle matching’ with the bristlecone pine data from Suess of 1978, indicating a preliminary dating using ¹⁴C [6].

Figure 1: The “bridge” of LateBC (localities Garry Bog 2, Dorsey Extended, Corlea) to BelfastAD (locality Teeshan) via Carlisle (Northern England) and Southwark (London) with the t values given by M. Baillie [13]

To evaluate the West German oak chronology of Ernst Hollstein (Trier), Larsson digitized Hollstein’s year-ring panels and verified them for internal consistency, with positive results except for a few samples, which were then not included in the collection. Hollstein’s chronology has two weak spots, described by him, and caused by lack of trunks: the “migration period gap” around the 4th century and the “Carolingian gap” around the 8th century (see fig. 2.). Because of the good cross-correlation with South English chronologies, the “Carolingian gap” can be bridged by these. Simultaneous to the “migration period gap”, however, the English chronologies also have gaps. The conclusion of the investigations by the Larssons was that Hollstein’s chronology is correct from 410 CE until 1974; also his Roman chronology from 546 BC to 250 AD was safe (which I write with different eras, Anno Domini and Before Christ, to indicate that it is “floating” with respect to later chronologies based on our Common Era and does not connect to them). In the period from 250 to 336 AD, the Larssons suspect an error in the data, but they cannot verify it without access to the raw data [6; 11]. Unfortunately, the German institutes refuse to disclose their raw data.

The South German oak chronology of Bernd Becker is only available as a mean value curve that “cross-correlates directly with the Hollstein and the South English chronologies” [6; 12]. It has gaps in the same places. The raw data is kept secret as well. A few collections of local data got onto the Internet through a leak and could be evaluated by the Larssons. Also data from Dutch and French dendrochronology labs, as far as they were accessible, were studied, and it showed up every time that the data from Roman-era wood could be synchronized with each other, but no connection to a chronology reaching back to the Early Middle Ages and continuing up to the present could be found [12].

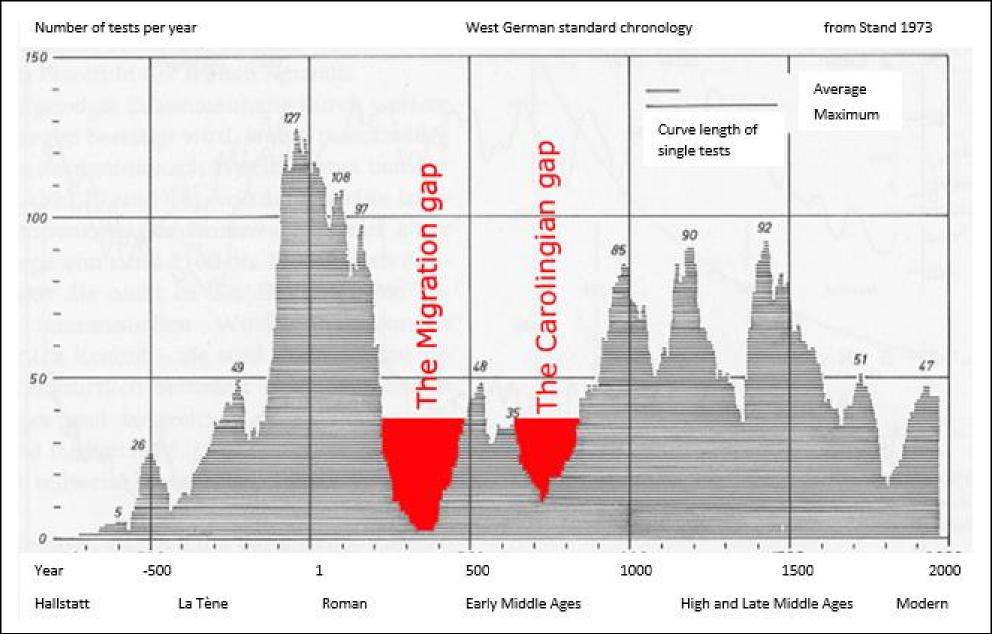
Figure 2: Plot of sample-density against time for the Central European oak chronology, with the Larssons' markings of the gaps [14]

The Dendrochronological Time Leap

In 2010, the Larssons compared partial chronologies from Hollstein’s collection with each other, and found a highly significant correlation (with t between 5.8 and 7.8) of Hollstein data for the years 401 to 543 CE with those of Hollstein data for 194 to 336 AD. Here a ‘time leap’ of 207 years seems to reveal itself – or a serious error in Hollstein’s data! [6] The results were posted on the Internet on 21 September 2010 [15].

They then constructed from the Northwest European Roman-era oak chronologies available to them, including the matching LateBC collection, a coherent chronology that reached almost 1,500 years from 1155 BC until 328 AD, and compared this with the Finnish pine chronology. The chronologies did not at all fit together at the conventionally expected time, 328 CE, or in the years around that date. Instead, the Larssons found a mathematically-significant synchronization point at 328 AD = 546 CE, that is, a shift by 218 years with respect to the conventional dating [16]. The diagram in fig. 3 shows the t-scores from the comparison for all years from 1155 BCE to 2004 CE. If you move the last year of the Roman-era oak chronology into the respective year in the Finnish pine chronology, the comparison of both chronologies yields the displayed t value. Around 328 CE the t-values are between 1 and just over 3. As for the year 546 AD, a t-value greater than 6 is obtained, 218 years later than the conventional dating. (There is a second peak with a t-score just over 5 in 1721 CE, but this is beyond any acceptable fit. Furthermore, another value, ‘skeleton Chi²’, has to be considered that here is very low and thus an incorrect fit, while this measure is high at 546 CE.) [17] The collections included in the Roman-era oak chronology (LateBC, North England, South England, London, North East France and the Hollstein data) each separately compared with the Finnish pine chronology show the same offset of 218 years compared with the conventional dating [16]. Another attempt, with more meticulously-selected sample collections reaching from 1155 BC to 315 AD, again yielded an offset by 218 years to 533 AD for the best synchronization point [18], see fig. 4. An analysis using data blocks having a length of 350 years at intervals of 30 years showed that the result is consistent over the entire length [19] – in every case there is one synchronization point with a 218 years offset.

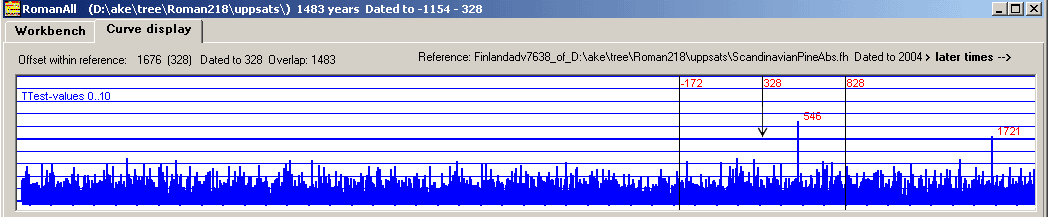
Figure. 3: t values for the entire range of the North West European Roman-era oak chronology compared with the Finnish pine chronology [20]

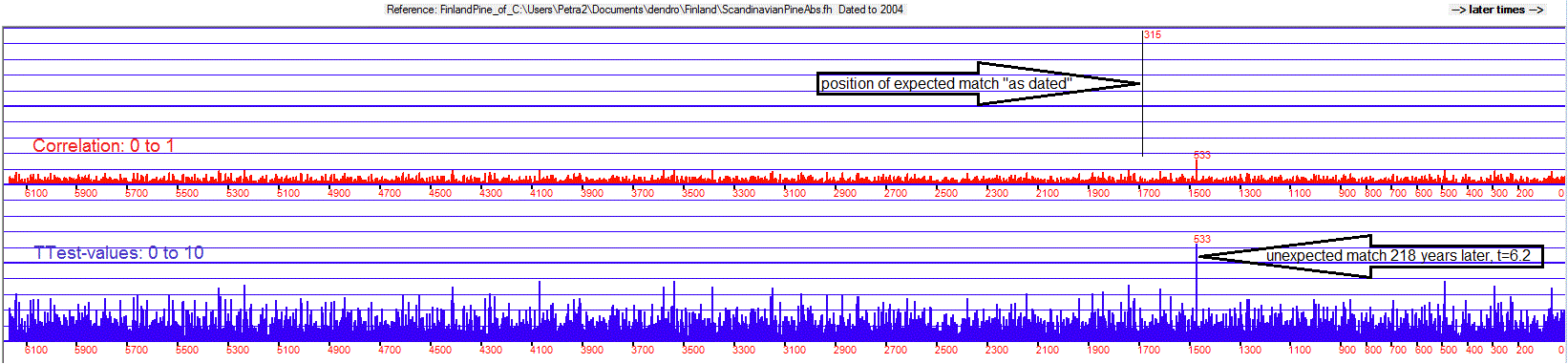
Figure 4: “Correlation coefficients and t-values for all possible positions of RomanAll oak towards FinlandPine. Hollstein normalization used” [21]

This result is not yet a proof of a phantom time because the comparison made here took place between different species from different regions, but nevertheless it is a strong indication. Greater certainty would be obtained if it were possible to correlate the Roman-era oak chronologies with absolute Northwestern European oak chronologies (i.e. reaching back from today down until antiquity). But the Roman timber sequence ends at 328 AD and is already sparsely equipped with samples after 220 AD, so no significant synchronization points can be found. Only one oak chronology exists today that reaches far enough into the past, has sufficient overlap with Roman-era oak chronologies, and for which the raw data is available. This is BelfastAD from 25 CE, which unfortunately is only weakly correlated with the Roman-era chronologies. There are other long absolute German oak chronologies, such as the South German oak chronology. However, besides including sunken oak trunks from riparian forests, the latter also contain Roman timbers which would corrupt any possible synchronization with a Roman-era chronology. The disclosure of raw data by the dendrochronology labs is required for assessment!

Archaeoastronomical Examination of the Thesis

The above result was not expected and is far from being capable of refuting the phantom time thesis, but it is rather a strong indication of a leap in the annual count by more than 200 years (although not much more) [22]. The Larssons concluded that there obviously was a phantom time, but not as long as Illig hypothesized. Trying to support their thesis with independent methods the Larssons checked astronomical retro-calculations of ancient observations.

Pliny reported a solar eclipse exactly 15 days after a lunar eclipse and a solar eclipse 12 years before [23]. The conventional dating has 16 days between lunar and solar eclipses, and the lunar eclipse is only weak. The search for a corresponding triple of eclipses 218 years or more afterwards found:

• Solar eclipse (SE) 30 April 59 CE  15 May 291 CE, so 232 years later.

• Lunar eclipse (LE) 4 March and SE 20 March (16 days after LE) 71 CE  LE 12 September and SE 27 September (15 days after LE) 303 CE, that is, 232 years later.

Pliny dated the eclipses to consuls named by him. The bulleted text gives the modern retro-calculated dates and then after the “” sign the retro-calculated date proposed by the Larssons. Details of this discovery were reported by the Larssons on their website [24].

Plutarch [25] tells of a solar eclipse, which is dated by Stephenson and Fatoohi to 20 March 71 CE, because the eclipse of this date “is by far the most likely of the various possibilities and is indeed virtually certain” [26]. As Plutarch made no statement about the year or place of his observation, but wrote only “the other day”, the Larssons had some freedom and searched for a solar eclipse in the period from 45+232 to 120+232 CE (the conventional key data of Plutarch’s life plus the offset determined from Pliny’s data):

• SE 20 March 71 CE about 10:00 - 12:50 local time or 5 January 75 CE about 15:30 – 17:40 local time, both in Greece  17 July 334 CE about 12:00 to 15:00 local time in South Italy [24].

Assuming an offset of 232 years, this is, relative to Plutarch’s lifetime, closer to the time when he wrote his dialog. According to retro-calculations performed by NASA, this solar eclipse was an “annular” one and only visible in South Italy. In Chaeronea in Boeotia, where Plutarch lived, the 71 CE [27] and 75 CE [28] solar eclipses, as well as the other one dated to 334 CE [29] (within the recalculated lifetime of Plutarch), were all visible as partial eclipses, with 95%, 88% and 90% totality, respectively. Was this sufficient to see “many stars everywhere in the sky”, as Plutarch noted? I was not sure about that but Ossowki Larsson explained:

Plutarch does not mention the place of observation, and he does not give a personal account, but lets the Roman, Lucius, report. Here, the time (just after noon) exactly matches our retro-calculation for Italy. [30]

Also, for Hipparchus’ reports on a solar and a lunar eclipse, when shifting his accepted lifetime by 232 years, relevant eclipses can be found which fit better to his lifetime data (about 190 to 120 BC) than the conventionally-accepted eclipses [31], in his case towards younger years of life:

• SE 30 November 129 BCE totally at the Hellespont, partially in Alexandria  20 March 71 CE.

• LE 26 November 139 BCE, total eclipse  11 January 65 CE.

Therefore, if Hipparchus had lived from about 42 to 112 CE, he would have seen the two eclipses in his third decade of life, before becoming famous, rather than as an old man [31].

Things are different with Hero of Alexandria. Some historians dated him to 150 BC, but because of a unique astronomical observation, he has been dated ‘absolutely’ to the middle of the 1^st century CE, namely because of a lunar eclipse that could be observed simultaneously in Rome and Alexandria, ten days before the spring equinox and in the fifth hour of the night in Alexandria. Although no year was specified, in the period from –200 to +300 CE the retro-calculations find a single lunar eclipse that suits Hero’s information, namely on 13 March 62 CE. This would make Hipparchus and Hero contemporaries – which was already presumed by some historians! [31]

A lunar eclipse at Arbela eleven days before the Battle of Gaugamela, won by Alexander the Great, was reported by Pliny and by Claudius Ptolemy. A lunar eclipse on 20 September 331 BCE dates the Battle of Gaugamela to 1 October 331 BCE. An alternative lunar eclipse 232 years later, on 6 October 99 BCE, would result in a date for the battle of 17 October 99 BCE. The hour-of-the-day given by Pliny, however, matches the eclipse of 331 BCE, but not that of 99 BCE; the hour given by Ptolemy does not match either [32].

Two fragments of a Babylonian cuneiform tablet mention a battle won by a king named Alexander, who conquered Babylon three weeks after the battle. The astronomical data on the tablet refer to a lunar eclipse retro-calculated to 20 September 331 BCE [33]. If this was the Battle of Gaugamela, it would be an indication against a leap in time!

Criticism of Archaeoastronomy

Now, various recalculations certainly can be done that lead to different ‘time shifts’, here demonstrated with reference to examples presented by the Larssons (all data in CE):

Hans Erdmann Korth [34] and Mario Arndt [35] provide many more examples of their respective favorite time leaps of 300 or 781 years. From this we must conclude that the length of the “phantom time” or the “dichotomy” (Korth) cannot be proved from astronomical precision-fit retro-calculations of eclipses alone. Arndt “initially assumed a chronology error in Europe of exactly 700 years” until he realized that many Roman eclipses up to about 400 AD showed matching duplicates at an offset of 781 years [36].

Korth found again and again, in the time span from antiquity to the Early Middle Ages, historical narratives which had a corresponding repetition at an offset of mostly 300 years. He searched for and found different scientific confirmations. For many reports of eclipses from Diodorus to Gregory of Tours, he found matching duplicates of conventional retro-calculations with an offset of exactly 300 years minus 46 days. In the ¹⁴C calibration curve, he demonstrated a plausible 300-years leap. Using data from ice-cores he identified errors in the dendrochronology.

The Larssons, on the other hand, found for Pliny’s solar and Gaugamela’s lunar eclipses an equally plausible offset of exactly 232 years plus 16 days [37]. Arndt in turn found a multiple re-occurring exact offset of 781 years plus 5 days [38].

How do such different interpretations emerge? Of course, eclipses occur periodically and are repeated in multiples of draconic and synodic months [39]. Already the ancient Babylonians knew this and could foretell eclipses. But to tell exactly where particularly solar eclipses could or would be seen required complex calculations that really only became possible with today’s methods and tools – that is, with computers.

Additionally, the possibility cannot be excluded that even those ancient reports which seem to match modern retro-calculations most suitably do not describe observations, but are based on calculations only. They might also have been ‘improved’ by later copyists to fit more precisely into their ideas of the historical sequences. The Larssons notice that every single report could be a fictional observation because the antique astronomers had the ability to anticipate and compute the described events. Therefore, the 232-year time leap hypothesis must remain a hypothesis forever [40]. But dendrochronology shows clearly that there must have been a leap in the counting of the years of slightly more than 200 years. Astronomical events found so far with an offset of 232 years fit consistently to this result [ibid].

About the Difference of 14 Years

The dendrochronological and astronomical time leaps differ by 14 years. That would mean that the dendrochronological data is dated 14 years too young within Roman history. The tree-ring chronology of the Roman era had been initially calibrated at the Rhine bridge in Cologne which was completed in the year 310 AD, as was known from ancient texts: therefore the youngest tree-ring of the bridge timbers could not be younger. With the chronology thus derived, the Roman military camp at Oberaden was dated to 38 BC [41]. From the coin finds and other archaeological findings, the camp was used in the time from 12 to 9 or 8 BC. This forced a displacement of the chronology by 27 or 26 years towards the present [42]. Hollstein confirmed the displacement by 27 years, and thus accepted that the Rhine bridge in Cologne was now given a construction date of 336 AD, though the panegyric attributed it to the year 310 AD [43].

By pushing Roman chronology forward by 14 years in relation to Roman dendrochronology, a part of this correction is made up again. The Larssons so see Tacitus rehabilitated: he was accused of having incorrectly dated the creation of Carlisle to 79 AD to favor his father-in-law, Agricola. Subtracting these 14 years, the first wooden fort was built in 58/59 AD, was repaired several times from 68 to 70 AD, and was extensively rebuilt from 79 to 81 AD, so Tacitus’ statement does not meet the original foundation, but this extensive reconstruction [44].

That the re-dating of a founding pillar from 71 to 57 AD would facilitate the interpretation of Tacitus’ report on the occupation of the Moselle bridge in Trier by insurgent troops, and its recovery by commander Cerealis in 70 AD [45], is not persuasive to me, as I presume that the assignment of the construction of the bridge to the time of Emperor Vespasian [46] was deduced from the dendrochronological dating of the wooden post. Tacitus’ report would yet have no problem with Hollstein’s dating. Then the old bridge from 17 BC was occupied and recaptured in 70 AD, and its possible damage would have been the reason for the reconstruction [ibid] or the repair [47]. Heinen noticed that former historians had assigned this bridge construction to the time of Emperor Claudius, but “according to the evidence of dendrochronology” [48] this assignment had to be given up. The 14 years do not bring the new bridge back to Claudius, but into the time of Nero.

Mechthild Neyses-Eiden claims, in relation to the early Roman military camp on the Petrisberg next to Trier: “Despite their small size, these timbers can be dated securely to the year 30 BC. The construction date of the military camp, 30 BC, which had been determined dendrochronologically, fits exactly to the political events in this time. […] From written tradition, we know that the Roman general, Nonius Gallus, had defeated the insurgent Treveri and their allies in 30/29 BC, and was acclaimed imperator [an honor given to a particularly successful general by his troops] in the year 29 BC.” [49]

Ossowski Larsson comments on this:

The dating of the Roman military camp on Petrisberg was carried out by Ernst Hollstein. The samples have 28 to 66 annual rings, and I believe Hollstein has solved the puzzle. At any rate, his mean-value collection matches significantly his Roman master-curve with the given dating. We do not have access to raw measurement series [but] with our correction this would be 44 BC [50].

The first Roman pile bridge over the Moselle was dated to 18/17 BC based on an “investigation of piles that were recovered in 1963 from the Moselle” [51]. With the Larssons’ readjustment of the Roman timbers, the construction of this bridge would come in the years 32/31 BC, just before or in the middle of the above campaign of Nonius Gallus against the Treveri. On the one hand this would furnish a motive for bridge construction but, on the other hand, it would impose difficulties.

According to Heinen:

The wooden sheet piling around the stone pillars [still today carrying the bridge] [...] can be dendrochronologically dated to the years 144 to 152 AD [52].

Neyses-Eiden dates it to the years “144-157 AD under Emperor Antoninus Pius” [53]. This assignment also appears to be derived from the dating of the wood. With the Larssons’ readjustment, construction of the new bridge would have begun under Emperor Hadrian in 130 AD; ultimately, the construction would still have been completed under Emperor Antoninus Pius.

Of interest in this context is the mineral spring, which is popularly referred to as “Römersprudel”. The interest is not so much because of the Roman-era timbers, which can be re-dated without problems from 81, 111 and 141 AD [54] to 67, 97 and 127 AD, but rather because of the “Bronze Age” spring frames, which were dated to 1969 BC and 1553 BC, incredibly early and with a still more incredible distance of over 2,000 and more than 1,600 years to the Roman frames found directly above them. They fit securely well to a corresponding oak chronology, but this “contains some gaps on the way to modern times” [50].

In an article in the magazine, Zeitensprünge [55], I published a table that listed “Selected monuments and archaeological features” in Trier with their respective datings. Only a small part of this dating was dendrochronologically determined and would have to be adapted to fit to the difference.

With the exception of the military camp on the Petrisberg, whose construction-details would now have to be re-determined, this 14-year shift fits perfectly to the ancient history of Trier. Construction of the third Moselle bridge would then have begun under Emperor Hadrian and would have been completed under Antoninus Pius.

Caesar’s Comet

With the hypothesis of a shift of the Roman period in the direction of today by 232 years we arrive at the conclusion that Julius Caesar was murdered in the year 189 CE. The majority of historians adhere to Octavian’s view, that the comet (sidus Iulium) had been observed during the sports events (held on the occasion of Caesar’s funeral) in July 44 BC, while others stick to the numismatic findings, showing coins of Caesar with his ‘star’, and advocate early 44 BC, or even the year 45 BC. Two hundred and thirty-two years later, if you search in the years 189 and 188 CE, you will find the comet Swift-Tuttle in the year 188 CE = 45 BC. The Larssons conclude:

To sum up, the Astr 188 [astronomically dated CE] apparition of Comet P/Swift-Tuttle seems to be a hot candidate for Caesar’s comet. It is bright and large, visible in the right celestial location over Rome and appears at the right time of the year (July, which is Caesar’s birth month and named in his honor). Its appearance a few months before Caesar’s death resolves the discrepancy between numismatic evidence and written (later) sources: it was probably Caesar himself, and not Octavian, who first used a star to promote the divinity of the Julian family. [56]

So it was written on the Larssons’ Cybis website, in June, 2015. As a published idea this is really new. However before them Korth had already claimed the comet Swift-Tuttle to be Caesar’s comet, to a small circle [57]. Korth is a natural scientist, and he does not rely on calculations done by others, but does the calculations himself. From many comparisons of scientific data (from ice-cores, dendrochronology and ¹⁴C data, which are – in his view – not consistent with each other, and which can only be reconciled with each other after recognition of different 300-year leaps for dendrochronology and ¹⁴C, respectively, as well as eclipse reports which have to be newly recalculated according to this finding) he has been convinced for quite some time that 750 AUC (ab urbe condita) = 300 CE. From certain inconsistencies in the biography of Augustus compared to other contemporary reports, he finally concludes that Caesar was murdered in 264 CE = 36 BC. From the three recent observations of comet Swift-Tuttle (1737, 1862, 1992 [58]), and ignoring the ancient Chinese data as well as the NASA reckoning (which is based on the assumptions that the ancient Chinese observations were, in fact, both Swift-Tuttle, and secondly that they were correctly synchronized with our CE chronology), he calculated a possible (for him, probable) appearance of Swift-Tuttle in 264 CE – without, however, disclosing his calculation – and so a time shift (which he calls “dichotomy”) by 44+264 = 308 years. (These 308 years concern here only the death of the first ‘Caesar’; the death of the first ‘Augustus’ he sees more likely at 306/307 CE, thus ‘only’ 307 minus 14 = 293 years later.)

Ossowski Larsson entered the discussion at that time and quickly found out that the date of 188 CE given by Yau et al [59] for an appearance of Swift-Tuttle corresponded exactly to her and her husband’s concept of a 232-year discrepancy. Subtracting 232 years, the Larssons arrived at 45 BC for the appearance of the Sidus Iulium (see above).

Delta-T

When retro-calculating ancient astronomical observations, the value of delta-T (ΔT) must be considered. Delta-T characterizes the assumed change in the ratio between the speed of Earth’s rotation, which is supposed to slow down in the long term, and the speed of the Moon’s orbiting of the Earth, which is supposed to accelerate over time, while its angular speed is also slowing down, but not so fast as the speed of Earth’s rotation. This means that, in times long past, the Moon moved faster than it does today through the sky, in relation to local time on Earth. Current values of ΔT are calculated as the difference between Terrestrial Time (TT), which is employed to calculate the movements of the celestial bodies, and the observed Universal Time (UT). Historical values of ΔT are estimates which have to be assumed in order to bring the retro-calculations of celestial phenomena (especially eclipses, but also planetary or cometary positions or their risings or settings) into correspondence with the ancient reports of observations [60]. These assumptions are, of course, based on the conventional chronology. If this is doubted, then the historical values of ΔT must be reasonably re-estimated for the events of concern, to be able to rule out the suggestion that the retro-calculated events were possibly not visible at the reported place of the observation. For readers who can read German, a deep criticism of the delta-T-curves is available from Arndt [61]. The Larssons reason that the Chinese observations are correctly dated and therefore provide correct ΔT values.

Who did it, and Why?

The Larssons suspect the time leap originated in one of the numerous antique and late antique inventions of chronologies and eras [62]. These calendar systems are all carefully designed. The Alexandrian monk Annianus selected as the starting point of his era the year of the Birth of Christ to be 5501 AM/A (Anno Mundi according to Annianus), and he selected the year of the Passion as 5533 AM/A so that the Easter moon (14^th day of the moon) fell on Thursday, 22 March, the Passion on Friday, 23 March, and the Resurrection on Sunday, 25 March, the start of the year 5534 AM/A [63]. In our calculation, this is the year 42 AD, and the Birth of Christ would be in the year 9 AD – twelve years after the death of King Herod of Judea, but fitting to the time “while Quirinius was governor of Syria” [64]! Panodorus of Alexandria adopts Annianus’ era, but he moves the beginning of the year – in accordance with the Egyptian civil calendar – back by seven months to 1 Thoth (29 August in the Julian calendar) [63]. Both have the year 1 AM as the starting point of the 19-year Alexandrian lunar cycles. Now we find that year 1 of Diocletian = 285 AD is the first year of the 305^th lunar cycle 5777 AM, and the year of the first consulship of Theodosius in 380 AD is the first year of 310^th lunar cycle 5872 AM. Mere coincidence, or the result of intentional reckoning?

In the Byzantine Empire in the 7^th century, the Byzantine Anno Mundi era (AM/B) was introduced [65]. This is constructed so that the Birth of Christ in 1 AD falls on 25 December 5510 AM/B, in the last year of the 290^th lunar cycle. According to Mosshammer [66], some ancient scholars even dated the Birth of Christ to the years 5506/07 AM/B, in the lifetime of Herod the Great. Dionysius Exiguus took over the Alexandrian lunar cycles of Panodorus, and he chose his year 1 AD/DE so that the new moon fell on 21 March, the Moon’s first new light on 22 March, and Easter full moon (“Luna XIV”) fell on 5 April, so for him this was “the ideal Easter moon” [67] – that is why his year 1 corresponds to the 17^th year of the Alexandrian lunar cycle. For him, year 1 of Diocletian is exactly 15 lunar cycles (285 years) after the year of the death of Herod the Great, which would therefore correspond to his year 0 AD/DE – though he did not know about the number zero! So much coincidence?

In the 7^th century, another Panodorus lived in Egypt [68]. Given the 232-years hypothesis of the Larssons, the “two Panodoruses” could well have been one and the same person, who was passed down to us in reports referring to two different eras [69].

We know Annianus and Panodorus only through excerpts of their works by George Syncellus. The latter also tells us that Panodorus used the “Astronomical Canon”, that is the list of the kings from the Almagest of Ptolemy, to position Jesus’ birth on the historical time axis [70]. The Larssons quote Panodorus via Mosshammer:

on the basis of the men whose genealogies have been traced in divine Scriptures from Adam up to Theophilus, destroyer of idols, the praiseworthy twenty-second archbishop of Alexandria, Egypt, and the two Libyas, I shall compute the chronology, and set forth the total number of years as 5904 – this so that both the heresiarchs and pagans, wise in their self-conceit, may find no basis of support in our divine Scriptures. [71]

To the Larssons, this very last sentence seemed to sound like a political program, raising the possibility that Panodorus could have manipulated the historical time-axis in order to make the Christian religion older and hence more venerable than the young Islam of that time [72]. For with the hypothesis that the year 412 AD/Rom in Alexandria is simultaneous with the year 644 AD/Byz in Constantinople, Arianism gains a very dynamic development, and 100 years after the first condemnation of Arianism at a Council, Islam emerged as the result of a theological controversy! The Christian Church reacted with a sharp persecution of all kinds of heresies: a strict consolidation of the Scriptures (canon of Athanasius 367 AD/Rom); a new definition of the time of the Easter festival; and a new chronology [73]. The Larssons saw the Almagest as a candidate for counterfeiting in the sense of an ‘adaptation’ to the new calculation of the years [74]. Then followed a discussion by the Larssons about Ptolemy and his critic Robert Newton; about the invention of the Diocletian era, also named ‘Era of Martyrs’; and about the dating of the chronicle of Hydatius [75].

Conclusion

The Larssons’ starting-point was the idea and then the project, to either falsify or verify Illig’s phantom time hypothesis using dendrochronology. The approach is welcome because its proceedings and its methods guarantee a timeline which is independent of historical traditions and can be independent of ¹⁴C measurements. They discovered some inconsistencies in the published long-term dendrochronologies, and they finally found, to their surprise, a temporal leap in the Roman-era oak chronologies of 218 years compared to conventional dating, and concluded from this a leap in the historic chronology of about the same extent. To secure the results, the raw data of the European oak chronologies is required, which demands the publication of this by the dendrochronology labs.

The absence of Roman timbers from the 4^th century in Hollstein’s collection is surprising, in particular those from the still-existing Roman central parts of the Cathedral of Trier. In the west façade, from the 11^th century, nine pieces of scaffolding wood were found which dated the construction time from floor to floor from “after 1042” until “after 1074” [76]. In the same way, the Romans are known to have embedded parts of the scaffolding in the walls, and cut it off at the edge of the wall using ax or saw when removing the scaffolding. However, Neyses-Eiden in her small treatise does not mention any wood from the Roman part of the cathedral, and Hollstein has no piece of it in his collection.

To determine the length of the chronological leap, the Larssons searched for, and found, matching retro-calculations of ancient astronomical observations with 232 years of displacement from the conventional dating. Above, I justified the statement that retro-calculations of historical astronomical observations alone cannot prove a chronological leap, even though there are many with nearly the same results, because other retro-calculations show different chronological leaps, or even no leap at all. However, together with a chronological leap obtained from an independent scale, as given by tree-rings, retro-calculations can lead to the adjustment of the chronological leap. This results in a 14-year difference between the Roman-era oak chronologies and the chronological leap inferred from archaeo-astronomical calculations. The timbers from the Rhine bridge in Cologne, built according to the panegyric in 310 AD, come from 336 AD to 322 AD, thus again closer to the historical date, but not reaching it. On the other hand, the timbers of the military camp at Oberaden come just after 26 BC, and thus again fall out of their numismatic context of 12 to 8 BC: both re-datings do not fit the historical records, and possibly the chronological connections of the woods of the Rhine bridge in Cologne are not correct, neither upwards nor downwards. However, these assumptions can only be checked when access to the raw data of the dendrochronological examinations becomes possible.

The length of the time leap remains controversial. Other chronology critics, some of whom have been dealing with the subject for more than two decades, are pleading for a longer time leap because of the lack of archaeological finds. Illig, who first discovered the time leap in the Early Middle Ages, assumes 297 years [77], and so do most of the authors in his magazine, Zeitensprünge, or they assume around 300 years. There also exist theses with substantially longer time leaps (Arndt, Heinsohn) [78]. The dendrochronological leap of 218 years was discovered by comparisons across tree species and across regions. Here again, certainty can only be obtained from the raw data of Western and Central European oak chronologies.

The assumption of the Larssons, that the time leap would only concern Rome and Northwest Europe, as well as Alexandria, but not Constantinople, is astonishing, given that archeological finds from the Early Middle Ages are missing in the Byzantine Empire, as they are in Rome, and in Trier, and elsewhere [79]. To find the chronological position of the Byzantine world and its predecessors, the olive trees and Lebanon cedars should be consulted.

After all, the Larssons not only published their results on their website, but fortunately uploaded their studies on academic platforms such as ResearchGate. It is to be hoped that someone who understands the matter will take on the task “to finally disprove this nonsense”, and will come up with new insights.

Notes and References

1. Lars-Åke Larsson & Petra Ossowski Larsson, Cybis Dendrochronology, http://cybis.se/forfun/dendro/index.htm (with links to further pages).

2. Wikipedia, “Student’s t-test”, https://en.wikipedia.org/wiki/Student%27s_t-test.

3. Petra Ossowski Larsson, “Wie man eine Referenzkurve baut”; several e-mails from 25 July until 3 August 2013, in a debating circle grouped around Gunnar Heinsohn, in which the author participated.

4. P. Ossowski Larsson, “Waldbrände”; e-mail from 20 July 2013.

5. P. Ossowski Larsson, “Willkommen”; e-mail from 27 July 2013; written in German, translated by the author.

6. P. Ossowski Larsson, “Die europäische Eichenchronologie - Irland und England”; three e-mails from 4 to 8 August 2013.

7. L.-Å. Larsson & P. Ossowski Larsson, An Irish tree ring chronology, 2010, http://www.cybis.se/forfun/dendro/hollstein/belfast/.

8. Robert M. Porter, ‘Recent Problems in Dendrochronology’; in: Peter James & Peter van der Veen (editors): Solomon and Shishak - Current perspectives from archaeology, epigraphy, history and chronology; Proceedings of the third BICANE colloquium at Cambridge 26–27 March 2011; British Archaeological Reports (International Series) 2732, Oxford, 2015; 225-233.

9. R. M. Porter, ‘Recent Egyptian Carbon Dating Projects and Dendrochronology’; in Chronology & Catastrophism Review 2015:11; pp. 2-6.

10. Ibid, p. 5.

11. L.-Å. Larsson & P. Ossowski Larsson, In search for a correct and continuous middlemiddlemiddleMiddle European Oak chronology, 2013, http://www.cybis.se/forfun/dendro/hollstein/.

12. L.-Å. Larsson, Retrieving some Becker data, 2008, http://www.cybis.se/forfun/dendro/hollstein/ → ‘Retrieving some Becker data’.

13. Larsson & Larsson, 2010, op. cit. [7, diagram], diagram1; original from Michael Baillie, A sliceslicesliceSlice through time - dendrochronology and precision datingTime - Dendrochronology and Precision Dating, Routledge, London, 1985, p. 40, fig. 2.5; also displayed by Porter, ref. op. cit.. 9, p. 5, fig. 3.

14. L.-Å. Larsson & P. Ossowski Larsson, Merging Hollstein curves, 2010, http://www.cybis.se/forfun/dendro/hollstein/ → ‘Merging Hollstein curves’; original from Ernst Hollstein, Mitteleuropäische Eichenchronolgie, Zabern, Mainz, 1980, Abb. 10.

15. L.-Å. Larsson & P. Ossowski Larsson, The ambiguous match in the Hollstein chronology, 2010, http://www.cybis.se/forfun/dendro/hollstein/ → ‘A very ambiguous match!!!’.

16. P. Ossowski Larsson, “Die absolute skandinavische Kiefern-Chronologie”; two e-mails from 10 August 2013; cf. ref. 11.

17. Larsson & Larsson, 2014, op. cit. [11]; for details →’Trying to date Roman time’.

18. L.-Å. Larsson & P. Ossowski Larsson, Dendrochronological dating of Roman time, 2014; published on Academia.edu> and Researchgate.net, 2015, pp. 22-24.

19. Larsson & Larsson, 2015, op. cit. [18], p. 23.

20. Larsson & Larsson, 2014, op. cit. [11], 2^nd diagram.

21. Larsson & Larsson, 2015, op. cit. [18], pp. 22-24, fig. 7.

22. L.-Å. Larsson & P. Ossowski Larsson, Astronomical dating of Roman time, 2016; published on Researchgate.net, 2016; p. 2.

23. Pliny the Elder, Historia Naturalis (Natural History), Book 2, xxxvii

24. L.-Å. Larsson & P. Ossowski Larsson, The validity of the European chronology - An astronomical approach to a wild idea, 2010, http://www.cybis.se/forfun/dendro/hollstein/ → ‘An astronomical approach’.

25. Plutarch, De facie quae in orbe lunae apparet (On the face on the moon).

26. F. Richard Stephenson & Louay J. Fatoohi, “The Total Solar Eclipse Described by Plutarch”; Histos 2, 1998, pp. 72-82; http://research.ncl.ac.uk/histos/documents/1998.04StephensonandFatoohiSolarEclipse7282.pdf.

27. http://eclipse.gsfc.nasa.gov/SEsearch/SEsearchmap.php?Ecl=00710320.

28. http://eclipse.gsfc.nasa.gov/SEsearch/SEsearchmap.php?Ecl=00750105,

29. http://eclipse.gsfc.nasa.gov/SEsearch/SEsearchmap.php?Ecl=03340717,

30. P. Ossowski Larsson, personal message to the author.

31. P. Ossowski Larsson, Ancient astronomers: Hipparchos, Hero and Geminos, 2010, http://www.cybis.se/forfun/dendro/hollstein/ → ‘Ancient astronomers’.

32. Larsson & Larsson, 2016, op. cit. [22], p. 6 and pp. 29-33.

33. Ibid, p. 6 and p. 34.

34. Hans Erdmann Korth, 68 Eklipsenberichte der Antike, 2013, http://www.jahr1000wen.de/jtw/Eklipsen-Hist.html.

35. Mario Arndt, Astronomie und Chronologiekritik, 2014, http://de.geschichte-chronologie.de/index.php?option=com_content&view=article&id=132:astronomie-und-chronologiekritik&catid=35:2008-11-15-18-09-31&Itemid=121.

36. M. Arndt, “Astronomie und Delta-T”, 27.12.2014, e-mail to the author.

37. Larsson & Larsson, 2016, op. cit. [22], p. 6.

38. Arndt, op. cit. [36], Tab. 1, “Römische Finsternisse der Antike nach Starke [S. 251 ff.] bis zum Ende des 4. Jh. mit alternativen Datierungen”.

39. Wikipedia, Orbit of the Moon, https://en.wikipedia.org/wiki/Orbit_of_the_Moon; Wikipedia, Eclipse cycle, https://en.wikipedia.org/wiki/Eclipse_cycle.

40. Larsson & Larsson, 2016, op. cit. [22], p. 22.

41. Burghart Schmidt, Dating of Roman sites; in: Dendrochronology and Archaeology in Europe, Proceedings of a Workshp of the European Science Foundation( (ESF), Hamburg 1982; Mitteilungen der Bundesforschungsanstalt für Forst- und Holzwirtschaft, Hamburg, Vol. 141, p. 150; Schmidt erroneously wrote “38 A.D.”, which is obviously wrong, because a few sentences further he wrote about “shifting […] from 38 B.C.”.

42. Larsson & Larsson, 2016, op. cit. [22], p. 38; Schmidt, op. cit. [41]; L.-Å. Larsson & P. Ossowski Larsson, About the offset between astronomical and dendrochronological error, http://www.cybis.se/dendro/dendro-audit/offset-between-astronomical-and-dendrochronological-error/, (without year).

43. Larsson & Larsson, 2016, op. cit. [22], p. 39; Schmidt, op. cit. [41], p. 151.

44. Larsson & Larsson, 2016, op. cit. [22], pp. 40-42.

45. Ibid, p. 39.

46. Mechthild Neyses-Eiden, Holz erzählt Geschichte. Dendrochronologische Forschungen zwischen Mosel und Hunsrück ; Schriftenreihe des Rheinischen Landesmuseums Trier, Nr. 29, Trier, 2005, p. 15.

47. Heinz Heinen, Trier und das Trevererland in römischer Zeit; 2000 Jahre Trier, ed. by Universität Trier, Volume 1, Trier, 1996, 5th edition 2002, p. 120.

48. Ibid, “nach Ausweis der Dendrochronologie”.

49. Neyses-Eiden, op. cit. [46], p. 13, translated by the author.

50. P. Ossowski Larsson, “Re: 14 Jahre Differenz”; e -mail from 8 June 2013, translated by the author.

51. Neyses-Eiden, op. cit. [46], p. 14.

52. Heinen, op. cit. [47], p. 120.

53. Neyses-Eiden, op. cit., [46], p. 16.

54. Ibid, pp. 10-12.

55. Karl-Heinz Lewin, “Trierische Spätantike – Noch unchristlich oder schon Phantomzeit?”; in Zeitensprünge 24 (1), 2012, pp. 125-154, 127ff.

56. L.-Å. Larsson & P. Ossowski Larsson, Caesar’s comet – myth or reality, http://www.cybis.se/dendro/ancient-history/caesars-comet/, (without year, actually published 2015).

57. H. E. Korth, “Cäsars Komet - Historisch-kritisch 2.0 - Jesus in Echtzeit”; six e-mails from 31 May until 29 August 2015.

58. Kevin Yau & Donald Yeomans & Paul Weissman, ‘The past and future motion of Comet P/Swift-Tuttle’; Mon. Not. R. Astron. Soc. 266, 1994, pp. 305-316; http://mnras.oxfordjournals.org/content/266/2/305.full.pdf+html .

59. Ibid, p. 309, Table 3. “Osculating orbital elements for comet P/Swift-Tuttle”.

60. Wikipedia, ‘ΔT’, https://en.wikipedia.org/wiki/%CE%94T; NASA, ‘Delta T (ΔT)’ , https://eclipse.gsfc.nasa.gov/SEcat5/deltat.html .

61. Arndt, op. cit. [35], chapter “Wie kommen die Delta-T-Werte zustande?”

62. Laurence Dixon, ‘When and Why they Changed the Calendar – From Tiberius to Bede’; in Chronology & Catastrophism Review 2012, pp. 16-24.

63. Alden A. Mosshammer, The Easter Computus and the Origins of the Christian Era, Oxford Early Christian Studies, Oxford University Press, 2008, 200f.; Mosshammer consistently writes ‘Annianus’, and the author follows him, though others write equally consistently ‘Anianus’, e.g. Wikipedia (see that name).

64. Luke 2,2; cf. Wikipedia, ‘Census of Quirinius’, https://en.wikipedia.org/wiki/Census_of_Quirinius.

65. Wikipedia, ‘Anno Mundi’, ‘Adoption of the Byzantine era’,
    https://en.wikipedia.org/wiki/Anno_Mundi#Adoption_of_Byzantine_era .

66. Mosshammer, op. cit. [63], p. 29.

67. Ulrich Voigt, “Über die christliche Jahreszählung” (On Christian counting of years), in Zeitensprünge 17 (2), 2005, pp. 420-454, pp. here 429, 447, 453.

68. Mosshammer, op. cit. [63], p. 359.

69. Larsson & Larsson, 2016, op. cit. [22], p. 18.

70. Ibid, p. 17.

71. Ibid, pp. 18/19, from Mosshammer, op. cit. [63], pp. 368/69, emphasis given by the Larssons’ text.

72. Ibid, p. 19.

73. Ibid, p. 18.

74. Ibid, p. 19.

75. Ibid, pp. 19-21.

76. Neyses-Eiden, op. cit. [46], pp. 24-25.

77. Heribert Illig, Hat Karl der Große je gelebt? Mantis, Gräfelfing, 1994, p. 20.

78. Arndt, op. cit. [35]; Gunnar Heinsohn, The Creation of the First Millennium CE, 2013, http://www.q-mag.org/gunnar-heinsohns-latest.html#tVZgrquO, http://www.q-mag.org/_iserv/dlfiles/dl.php?ddl=gunnar-creation-of-the-1st-millennium-new16-11-2013.pdf.

79. Richard Krautheimer, Early Christian and Byzantine Architecture, Yale University Press, 1986, e.g. p. 284: “In Constantinople [...] the evidence for church building [in the Early Middle Ages] is nil”.

Karl-Heinz Lewin, Haar: k-h-lewin@t-online.de

Copyright © Karl-Heinz Lewin, 2017

First published in: Chronology & Catastrophism Review 2017:3, pp. 7-16

Copyright © Journal of the Society for Interdisciplinary Studies, 2017